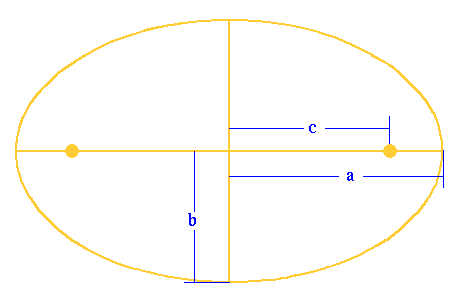

If this ellipse described an orbit of a planet, then the Sun would be located at one of the foci and the path of the planet would be along the ellipse. the eccentricity of the ellipse is given by:
e = c/a .
As shown in the table below, the orbits of the planets in our solar system are nearly circular, with eccentricities that are very small. Within the inner solar system, Mercury has the highest orbital eccentricity, 0.2. In fact, only Pluto, the outermost known planet, has a higher eccentricity. All other orbital eccentricities within our solar system are less that 0.1, with most having eccentricities of only a few percent. This means that the orbits are nearly circular, with the two foci of their orbits being only very slightly separated. In fact, a circle is a degenerate forn of an ellipse, with
a = b = r
and an eccentricity of 0. [For comparison, the eccentricity of the ellipse shown above is 0.79.]
| Planet | Orbit Radius | Eccentricity |
|---|---|---|
| (km) | ||
| Mercury | 5.79 × 107 | 0.206 |
| Venus | 1.08 × 108 | 0.007 |
| Earth | 1.50 × 108 | 0.017 |
| Mars | 2.28 × 108 | 0.093 |